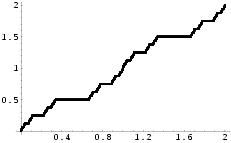
It consists of two copies of the Lebesgue function which is constant on the complement in [0,1] of the usual Cantor set.
These are some of the graphics that I have been drawing using methods for drawing the Douady-Earle extension of homeomorphisms of the circle and, more generally, degree 1 monotone mappings of the disk. The mathematics is partially a result of collaborations with Taiping Ye and Sudeb Mitra, along with a key contribution by Jack Milnor (the references can be found in my List of Publications). The computations were performed on computers, both individual and a cluster, partially funded by the National Science Foundation.
Here's the boundary function that is wrapped around the unit circle.

It consists of two copies of the Lebesgue function which is constant on the
complement in [0,1] of the usual Cantor set.
The image of concentric circles under the extension of the Lebesgue function is

The image covers the open unit disk. Hyperbolic half-planes bounded by arcs on which the boundary function is constant are mapped into the unit circle. The next image shows preimages of concentric circles. The inverse map is unstable near the boundary points which are images of nondegenerate intervals. The next image shows this instability.
